What is mathematics? How can we teach it more effectively? To answer these questions, let's first examine an analogy: What is money? How do we teach about money effectively?
The dollar sign ($) is a symbol of money in the United States. But the $ symbol itself is not money - money is something "behind" or "beyond" the $ symbol. The $ symbol is just one model of money. It is a symbol (just like numerals and signs), and symbols are considered Abstract models. There are several other models of money - such as coins, bills, or certain amounts of goods (one dollar's worth of cheese), time (one dollar's worth of tutoring), or services (one dollar's worth of babysitting). This variety of other models makes the Abstract model of the $ symbol more sensible, meaningful, and memorable. Note also that these other models are more interesting and motivating to children than just the $ symbol. As children gain experience "translating" from coins to goods to bills to time to symbols, this exposure occurs in highly connected and sensible ways that make it easier to work eventually with just the Abstract $ symbol alone.
If we want our children to work fluently with the Abstract models of money, we don't give them endless problems using the $ symbol. We provide them regular practice "translating" between the many models of money, because money is more than just a symbol.
Multiple Mathematical Models
Likewise, mathematics is not merely the Abstract model of written symbols like 2 + 7, sin 90 degrees, or /. There are quite a few models available - besides the traditional Abstract written symbols - to represent math facts, concepts, and procedures. The most common and important mathematical models are:
- The Real model: physical, real-world objects, such as five rabbits, or five pencils.
- The Musical model: the skip counting notions (discussed in a previous article).
- The Verbal model: the spoken word "five."
- The Concrete model: math manipulatives, such as an actual, physical arithmetic rod of 5 units (like Cuisennaire rods or Mortensen Math pieces).
- The Pictorial model: sketches, diagrams, and pictures. nnnnn or JJJJJ are Pictorial models of 5.
- The Abstract model: the traditional symbols of math, including the numerals 0 to 9, signs such as + and /, and other symbols such as pi and e. The Abstract model for the number of apples above is the symbol "5".
- The Written model: the written word "five" (distinct from the spoken word "five").
Some key action words for each model would be: Real : hold; Musical : sing; Verbal : say; Concrete : build; Pictorial : draw; Abstract : write or see (symbols); Written : write (words).
Teaching Translations
If we want our children to work fluently with the symbols of mathematics, we should not give them voluminous paperwork that deals primarily with just the Abstract model (as many math programs do), with a few pictures here and there and a token use of manipulatives. A far more effective approach is to provide children substantial experience with all the learning models along with numerous "translations" between those models. This approach engages more of the many senses God provided - and then connects them in a cohesive way.
Here are just a few examples of the many possible translations between models. These kinds of translations could be applied to almost any math topic (fractions, place value, negative numbers, etc.):
Using multiple models and doing math translations steers us clear of the biggest and most common mistake in elementary math: pushing kids into the Abstract model too quickly. Thinking abstractly is hard for young kids. But using other models that gradually and sensibly connect with Abstract models will build depth and understanding.
Putting it into Practice
Let's examine some practical ways to use this multiple model approach. The "whisper counting" notion in Figure 1 connects several different mathematical models in an almost seamless fashion [see also "Skip Counting," PHS #13, p. 14]. Figure 1 incorporates the Pictorial model of the rectangle diagram with the Musical model of actual singing, along with the Abstract model of the consecutive numerical Abstract symbols, and the Verbal model of the spoken words for skip counting. If actual arithmetic rods were also used, then the Concrete model is also being connected. In this case, there would be a high degree of consistency for children among:
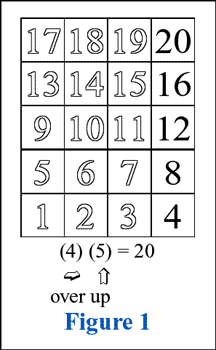
- what they say with spoken words,
- what they see with the diagram,
- what they build with the manipulatives,
- what they sing with the music, and
- what they read with the abstract symbols.
Another example of translations is the "over" and "up" notation in the rectangle model of Figure 1 [see also "Using Rectangles," PHS #14, p. 20]. Using the arrows (^ for "over" and for "up") promotes translational consistency between the Pictorial model (the arrows and the diagram), the Verbal model ("the 'over' amount is 4 and the 'up' amount is 5"), and the Abstract model notation of (4)(5) = 20, which leads eventually to the format for multiplication: 4 x 5 = 20.
From a design perspective, I personally doubt that our wise Creator ever meant for pencil-and-paper symbols to be the overwhelmingly dominant means of implanting mathematics into the minds and eyes of the elementary-age children whom He created. Paper-and-pencil symbols eventually? Absolutely! But the almost exclusive mode for grades K-6? Doubtful.
The use of multiple models to represent math draws upon the many senses and "information avenues" that God built into children. Moreover, the process of translating between models makes math sensible for kids, because there are solid, meaningful connections between what children see, say, build, write, draw, and sing. Your children don't like to write math? No problem for now - have them build, draw, see, say, and perhaps even sing their math activities, with some writing thrown in as a small part of their math lesson. I repeatedly remind my students: "Say what you see, write what you say. Say what you see, build what you say. Say what you see, draw what you say." You can make math that simple. It's a lot of fun, too, to see their eyes light up with understanding.
A final reason for using this translation approach is that it draws upon a wider range of learning styles. The three major learning styles (auditory, visual, and tactile-kinesthetic) are not completely fixed or unchanging for a child. Individual learning style preferences are affected by mood, time of day, appetite, sleep needs, and other factors. A math methodology that broadens a child's repertoire of learning strategies in a highly connected manner means that that child has a variety of learning tools to help him organize, retain, and recall the many math facts, concepts, and procedures being studied.
Manipulatives per se are not the key. A strong math methodology comes from using multiple models and doing translations between models, combined with connecting principles such as those in this series. Let's draw upon the fullness of what God made kids to be, letting them say, see, build, draw, write, and even sing math.
