 Math comes in lots of different flavors, all of which fall into either applied math or pure math. Applied math subjects were all invented to serve some other discipline. For example, calculus was invented as a tool for physics. The important thing to an applied mathematician is that he can get the result he wants from his mathematics. He is not all that concerned whether what he is doing is rigorously provable as long as it works.
Math comes in lots of different flavors, all of which fall into either applied math or pure math. Applied math subjects were all invented to serve some other discipline. For example, calculus was invented as a tool for physics. The important thing to an applied mathematician is that he can get the result he wants from his mathematics. He is not all that concerned whether what he is doing is rigorously provable as long as it works.
I overheard a classmate coming out of one of my more abstract pure math courses complaining, "What is this math good for anyway?" To a pure mathematician, this is a heretical question. Pure math is an end in itself. It doesn't matter if anyone has found a use for it yet. If it is logically rigorous and consistent, it's math and it's beautiful.
Geometry lends itself to both math approaches. It was invented originally as a tool for surveyors (the word geometry literally means "measuring land") and it can be learned entirely from an applied math point of view. The angles of a triangle add up to 180° whether or not you understand why. The Pythagorean Theorem holds for every right triangle whether you can prove it or not.
You never have to "do proofs" on a standardized test. You only have to be able to do the geometry problems and pick the right answer from the four or five possibilities. Once you learn the relationships between the parts of geometric objects and the formulas for various areas and volumes, you use those facts to solve problems. Throw in a little algebra and you have an answer. Geometry can be taught this way.
The advantage of this approach is you don't need a separate geometry course. You can pick up this amount of geometry between the lines of your Algebra I and Algebra II courses. The Saxon algebra courses widely used by homeschoolers have no separate geometry course for this very reason.
On the other hand, this "teach to the test" approach to geometry misses out on the true richness and enjoyment that is geometry.
Why Study Geometry?
So why should we study geometry? Number one, because most colleges expect students to have studied geometry in high school. Also, geometry is the starting course for every course sequence in math and the physical sciences. Take physics, for example. Calculus is a prerequisite for engineering physics. Trigonometry is a prerequisite for Calculus. Geometry is a prerequisite for trigonometry.
Connection to History
High school geometry is called "Euclidean Geometry," after a Greek named Euclid who lived in Alexandria, Egypt, around 325-265 B.C. He was the one to first systematize geometry and his system is still used to teach geometry now. It is mind-boggling to think that when you are doing geometry, you are studying a subject whose syllabus was designed by a man who lived 2,300 years ago. It's good for a kid's mind to be boggled every now and then. They need to realize that not every major invention and discovery was made this century
The five postulates of geometry as defined by Euclid are these:
- Any two points can be joined by a straight line.
- Any straight line segment can be extended indefinitely in a straight line.
- Given any straight line segment, a circle can be drawn having the segment as radius and one endpoint as center.
- All right angles are congruent.
- If two lines are drawn which intersect a third in such a way that the sum of the inner angles on one side (angles A and B in Figure 1) is less than two right angles, then the two lines inevitably must intersect each other on that side if extended far enough.
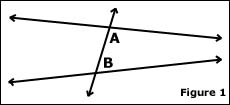
These postulates, with the possible exception of number five, are universally accepted and form the basis for all modern geometry courses. The rest of geometry follows from these five statements.
Geometry is Hands On
Geometry is the best form of math for a kinesthetic/tactile learner. These learners learn best using hands-on methods. They tend to comprehend things better if they can touch them.
Geometry has to do with the real world shapes of the objects around you. It involves a lot of drawing. You are always having to copy diagrams from problems or make up your own.
The most hands-on part of geometry is doing constructions. Using a compass and a straight edge (a ruler with no markings on it), you learn to draw all kinds of geometric objects. For example, you might be asked to draw a line perpendicular to another line through a given point on the line, or off the line. You may have to divide a line segment in half, or into a dozen equal pieces. You can copy an angle or a triangle. You can divide an angle in half, fourths, or eighths (but you can't divide it into thirds). A whole branch of mathematics has to do with what can be constructed this way and what can't and it's all hands-on fun.
note: If you are going to do constructions, get a draftsman's compass with a real point on it. The rubber point compasses they sell in school supply stores walk all over the paper. They are frustrating to use and you have to work hard to get accurate results.
Unfortunately, a lot of today's geometry courses skip over or at least barely touch on the art of geometric constructions. If your geometry program does this, there's no need to miss out. Just add the excellent, inexpensive Key to Geometry workbooks from Key Curriculum Press. They provide a constructions-based introduction to all of geometry.
Geometry Teaches Logic
What does everybody in public school use to scare you away from geometry? In geometry you have to do proofs - those dreaded proofs.
Proving things is what geometry is all about and once you get used to doing them, proofs are the most satisfying part of geometry.
Let's say your teacher tells you the angles of a triangle add up to 180° - how can you be sure? Pure mathematicians are from Missouri. They say, "Show me."
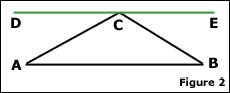
Okay. Take any triangle. Draw a line parallel to its base AB through the opposite vertex, C.
Once you have done that, the proof goes like this:
| Step |
Reason |
| m(<BAC) = m(<ACD) |
Alternate angles |
| m(<ABC) = m(<BCE) |
Alternate angles |
| m(<ACD) + m(<ACB) + m(<BCE) = 180° |
Three angles form a straight angle. Straight angles are 180° |
| m(<BAC) + m(<ACB) + m(<ABC) = 180° |
Substitute from first two steps |
There it is, the three angles of the triangle add up to 180°.
This proof uses a fact you already had proven, that if you cross two parallel lines with another line, alternate angles are the same size. It also uses the definition of a straight angle and a little algebra. And that's what proof is all about, using what you already know to prove new things that you can then use to prove more.
Perimeter, Area, & Volume
You are familiar with these types of problems from grade school. What is the perimeter of a triangle? Or what is the area of a square? In high school the shapes are more complex, so you have to divide them into shapes that you can solve and add up the results. You also have to learn the formulas for circumference and area of a circle, as well as surface area and volumes for solids like prisms, pyramids, cylinders, cones, and spheres. This is not a lot to memorize compared to subjects like history or biology which are nothing but memorization.
Expect to see this sort of problem on standardized tests.
Solving for X
And now, the other type of geometry problem you are likely to find on a standardized test. Given some of the measurements on a diagram, you will be asked to find the length of a given line, the measure of an angle, the area, the volume, etc. You have to know a few simple geometric formulas and theorems, e.g., "the three angles of a triangle add up to 180°," in order to solve the problem.
What Helps Are There?
You certainly aren't on your own when it's time to teach geometry. There is an abundance of curriculum and teacher's aids - so much it can be hard to choose.
Workbooks and textbooks. Just about every homeschool curriculum publisher has a geometry text. Possibly the most popular geometry textbook programs commonly used by homeschoolers are those published by A Beka and BJU Press.
DVD instruction. These programs go beyond textbooks by offering an instructor teaching and working problems on video. Accompanying textbooks or worksheets and answer keys are also included. Chalk Dust and Videotext Geometry fall into this category.
Software is interactive. It provides demonstrations and grades problems. The disadvantage of software is that you have to sit in front of the computer: not good for more than one kid at a time. Software geometry curriculum is available from a number of publishers, including Classmates and Grasp Math.
Online courses are also interactive, but add tutors as well so kids can email a teacher with their questions. The Apex learning online course also includes worksheets (with some constructions) that kids fax in for grading. Online courses tend to be more expensive
Review notes. The Quick Study guide set condenses all of high-school geometry into 10 colorfully illustrated laminated pages of definitions, postulates, and theorems. It's a handy, inexpensive way to improve, review, and keep on top of your geometry knowledge.
That's all there is to geometry: constructions; proofs; perimeters, areas, and volumes; and figuring the size of one thing given the size of another.
Once you have completed Algebra 1, Algebra 2, and Geometry, you're ready for advanced math, which will be our topic next issue.